Irreducible complexity. That phrase gets a lot of attention in the creation-evolution debate, or the accident-design debate. As well it should. Irreducible complexity shows that life did not come about by accident, but by design. Recently the advocates for “intelligent design” claimed vindication. Now it’s time to review the concept.

Irreducible complexity and what it means

Irreducible complexity generally means this: any system must be at least complex enough to function. The question is, how complex must it be? Charles Darwin himself admitted: if anyone could show that a biological system was too complex to come about by accident, his entire theory would fail.

Michael Behe made “irreducible complexity” a household phrase with his book, Darwin’s Black Box. His thesis: at last biochemistry and cell biology had found out what was in the cell and how the cell worked. Behe found that a cell needs a minimal set of subsystems to work. And to get that, everything had to come together at once.

Criticism from probability theory

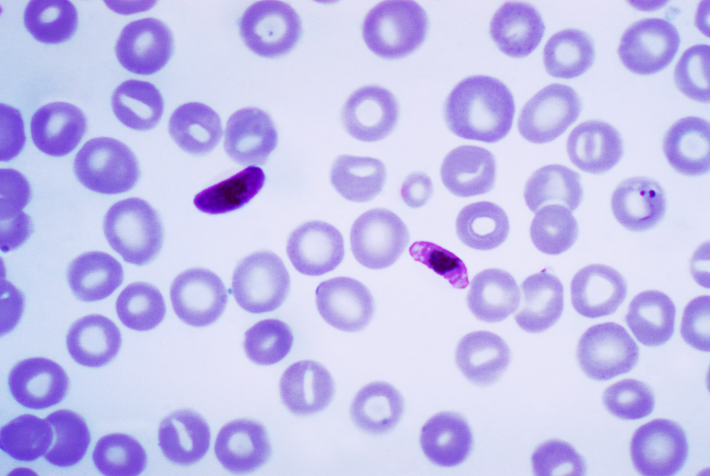
The classic Victor mousetrap. If any part of this system does not work properly, it will not catch any mice. Photo: Evan-Amos/Wikimedia Commons, released into the public domain

Behe’s detractors have always held that a system could go from non-living to living, and from simple to complex, step by step. Why does this matter? Because one computes the probability of step-by-step events differently from the probability of several events happening at once.

For instance: a club holds an election of officers. They must elect one each President and Vice-President. The club has ten members, five men and five women. What are the chances of electing an all-female (or both-female) ticket?

First the club elects a President. It has a fifty-percent probability of electing a woman President.

Next, the club elects a Vice-President. But the President cannot be a candidate for this second election. The chances of electing a woman Vice-President now are four in nine, not one in two. So the chances of electing a both-female ticket are 1/2 times 4/9, or 2/9. Twenty-two percent.

But that doesn’t tell the whole story. If a man wins election as President, the game is over. But once a woman wins election as President, the chances of getting a both-female ticket now double, to forty-four percent.

Now consider another problem: three men toss “odd man out” to decide who gets a prized seat in a cubicle office. What are the chances they will have to toss a second time?

Three men can toss three coins eight different ways. Two of these ways (all heads or all tails) decide nothing, so the men must toss again. The other six ways all have one coin landing differently from the other two. He who tosses that coin is the “odd man out.”

So the chances of tossing a tie game are twenty-five percent. And the chances of a deadlock go down with every toss they make from then on. After three tosses, they would almost certainly have a winner.

Now: every man’s chances of winning are exactly thirty-three and one-third percent. So: what are the chances that the same man would win at “odd man out” no matter how many “cubicle door prizes” they must award? Answer: any man has one chance in three of winning once. He has one chance in nine of winning twice in a row. And he has one chance in twenty-seven of winning a third time. Three point seven percent.

The statisticians now weigh in. Any time something keeps happening that has one chance in twenty, or fewer, of happening, something is making it happen. Or in this case: if one man wins three games of odd man out in a row, and this happens without fail every time those men have a round of tossing, he is cheating. Or, as the late Ian Fleming might have said: once is happenstance, twice is coincidence, and the third time it’s a cheat.

More broadly: game theorists live by a rule: the Law of Large Numbers of Trials, or the Law of Averages. This law says: Anyone who wins a game more often than his chances of winning would reasonably allow, is cheating. And over time, a player’s actual winnings must approach the calculated chances of winning. If they do not, the house should bar him from the gaming tables. Winning streaks always break.

Irreducible complexity is about saying life itself cheats at the cosmic roulette wheel that, evolutionists insist, was how life began and how life changes and becomes more complex. Why? Because life itself seems on an unbreakable winning streak. The evidence for that unbreakable winning streak is irreducible complexity. Certain life systems and subsystems are so complex, life had to be on a winning streak longer than any rational estimate to succeed.

Of Behe, Lenski, Dawkins, and others

Richard Lenski famously produced a strain of Escherichia coli that gained the ability, after several generations, of using citric acid as food. Evolutionists, like Richard Dawkins, jumped on this. In The Greatest Show on Earth, Dawkins wrote:

There is a comic sequel to this triumphant tale of scientific endeavor. Creationists hate it. Not only does it show evolution in action; not only does it show new information entering genomes without the intervention of a designer, which is something they have all been told to deny is possible (‘told to’ because most of them don’t understand what ‘information’ means); not only does it demonstrate the power of natural selection to put together combinations of genes that, by the naïve calculations so beloved of creationists, should be tantamount to impossible; it also undermines their central dogma of “irreducible complexity.” So it is no wonder they are disconcerted by the Lenski research, and eager to find fault with it.

Not so fast, wrote Casey Laskin at The Discovery Institute. As Behe himself wrote: the wild type of E. Coli already uses citrate internally. It’s getting citrate from outside the cell to the inside, that’s the problem. And: how did the successful strain solve the problem? It turns out the E. coli strain had to change, or “mutate,” twice to use citrate as food. And more generally:

If only one mutation is needed to confer some ability, then Darwinian evolution has little problem finding it. But if more than one is needed, the probability of getting all the right ones grows exponentially worse.

In other words: the game gets orders-of-magnitude harder to win with every new change a germ, or other life form, has to make. Hard enough, eventually, to say: It had to have help.

Four days ago (June 14), Michael Behe claimed vindication. At issue this time was how the parasite Plasmodium falciparum (the cause of the most dangerous form of malaria, or the classic “ague” known to mariners) might change itself to resist chloroquine. Sure enough, Robert L. Summers and his colleagues found out that P. falciparum must change itself at least twice to resist this drug. (Summers et al., “Diverse mutational pathways converge on saturable chloroquine transport via the malaria parasite’s chloroquine resistance transporter,” PNAS, 111(17):E1759–E1767, 29 April 2014. DOI:10.1073/pnas.1322965111) To quote their abstract:

We found that two main lineages of mutational routes lead to chloroquine transport via the chloroquine resistance transporter (PfCRT) and that a low level of chloroquine transport is conferred by as few as two mutations. However, the attainment of full transport activity is a rigid process that requires the mutations be added in a specific order to avoid decreases in chloroquine transport.

In other words: P. falciparum must take at least two steps, in the proper order, to resist the drug.

Two days later, Casey Luskin jumped on this. Her point: Richard Dawkins, and many other detractors of Michael Behe’s insight, now owe him an apology. Their whole point was that the Game of Life was easier to win than Behe gave it credit for. Not true.

Behe’s point was this: it probably takes one hundred sextillion generations of P. falciparum to resist chloroquine. It would take the square of that number to get something comparable to that, to give a creature a permanent survival advantage. But that would equal the total number of specimens that have ever lived.

Tell us again, Professor Dawkins, Dr. P.Z. Myers, and estate of Stephen Jay Gould, how easy the Game of Life is to win, without Someone picking winners. In fact, Myers had to admit, back in 2007:

Fair enough; if you demand a very specific pair of amino acid changes in specific places in a specific protein, I agree, the odds are going to be very long on theoretical considerations alone, and the empirical evidence supports the claim of improbability for that specific combination.

Dr. Myers, call your office! Have them get Dr. Summers on the line. Because that’s exactly what Dr. Summers found! Summers wasn’t looking to vindicate Michael Behe or anyone else. He was interested in only one thing: how to treat effectively a patient presenting with “the ague.” He was saying, “Take heart! It’s harder than you think for that parasite to resist our drugs. Hit them over and over again, don’t give them time to adapt, and you can beat them!” Or words to that effect, right in the abstract. But when he said that, he did vindicate the principle of irreducible complexity.

It’s hard enough, as a scientist looking for ammo against malaria found, for that bug to resist our arsenal of drugs. How much harder must it have been for that bug to put itself together in the first place. Especially when even the simplest germ has enough information, in the single strand of DNA that gives orders for its making and working, to fill ten Libraries of Congress.

All this is further proof that life is not “happenstance” or even “coincidence.” Life is a Divine Plan. Irreducible complexity shows this.

Reprinted from examiner.com

[subscribe2]